Ratio and Proportion

1. (d); Let present ages of Ramu and Somu be R and S respectively.
7R – 6S = 1 …..(i)

8R – 7S = – 4 ….(ii)

Multiplying equation (i) by 8 and equation (ii) by 7, We get 

56R – 48S = 8 ….(iii)

56R – 49S = – 28 ….(iv)

Subtract (iv) from (iii): S =36years

Shortcut:

One year ago → R:S ⇔ 6:7 ⇒S=7

Only option (d) has a number from which, if we subtract ‘1’, we get a number divisible by 7.

2. (b); 33% of A = 33 × A/ 100 =  55× B/100 = 55% of B

= 33 × A/100 = 55 × B/ 100⇒ A/B = 55/33

A:B⇔ 5:3

3. (a); 68% of two fifth of 550

= 68(2/5 * 550)/100 = 68*220/100 = 748/5 =149.6

4. (c); Let, number is ‘n’

(45n/100 )-24=48⇒ 9n/20 – 24 = 48

9n-480=48*20⇒ 9n = 960 + 480

n= 1440/9 =160⇒ 3/8 * n = 3/8 * 160 = 60

5. (a); 30% of the number = 190.8

1% of that number = 190.8/30

175% of the number = 1908/300 × 175=159×7 =1,113 

6. (c); 3/8 * 1000 =375⇒ 32% of (3/8th of 1000) = 32% of 375 = (32 * 375)/100 = 120

7. (c); Let the third number be n.
20% more than n = n + n/5 = (6n)/5

50% more than n = n + n/2 = (3n)/2

Ration of (6n)/5 and (3n)/2 = 6n/5 × 2/3n = 4:5

Shortcut
Let the third number be 100.

Then the two numbers are,

20% more and 50% more

⇓                         ⇓
120                     150

Ratio 120:150=4:5

8. (c); A: B: C:D⇒ 5:2:4:3

⇒ C gets 1 unit more than D.
Given, C gets Rs. 1000 more than D.
⇒1 unit = Rs, 1000 ⇒B’s share = 2 units

= 2 * 1000 = Rs. 2000

9. (b); 0.75:x::5:8

0.75/x = 5/8⇒ 5x = 6.00⇒ x=1.2

Shortcut

0.75:x::5:8 ⇒ 0.75 → 5 ⇒ 1 →0.15 ⇒8→1.20

10. (b); Three numbers = I, II, III

I + II + III = 98, I : II → 2:3, II: III → 5:8

10:15 ⇐ (2:3) × 5 ← (I : II) × 5

15:24 ⇐ (5:8) × 3 ← (II : III) × 3

I: II: III, 10:15:24, 10 + 15 + 24 = 49

Given I + II + III = 98, 98/49 = 2

⇒ 1 unit = 2

⇒ Second number = 15 × 2 = 30

11. (b); A : B = 5 :7

B: C = 6:11

So, we have to quantity B which to be equal in both case.
So, A : B = [5 : 7] * 6

B : C = [6: 11] * 7

So, A : B : C =  30: 42 : 77

12. (d); Let A =2x, B = 3x and C = 4x

So, A/B = (2x)/(3x), BC = (3x)/(4x) and C/A = (4x)/(2x)

⇒ A/B: B/C: C/A = 2/3:3/4:4/2 = 8:9:24

13. (b); 4³⁵/2⁵= 2^(2×2.35)/2⁵ =2⁷/2⁵ = 4/1

14. (a); x/y = 2/1, x²/y² =4/1

⇒ (x²+ y²)/(x²-x²) = (4 + 1)/(4 – 1)

(x²-y²)/(x²+y²) = 3/5

15. (c); 19(4x² – 3y²) = 12(2x² + 5y²)

52x² =117y² ⇒ 4x²= 9y²

x/y = 3/2

16. (a); Let (a + b) = 6k, (b + c) = 7k and (c + a) = 8k then, 2(a + b + c) = 21k

2*14=21k⇒ k = 4/3

So, (a + b) = 6 * 4/3 = 8

c = (a + b + c) – (a + b) = 14 – 8 = 6

17. (d); Given ratio = 1/2:2/3:3/4 = 6:8:9

So, Ist part is = 6/23 × 782 = 204 

18. (d); Let the original salaries of Ravi and Sumit be 2x and 3x.

Then, (2x + 4000)/(3x + 4000) = 40/57

3x = 34000

Sumit’s present salary = (3x+4000) = 38000

19. (b); A = 2/3 * B,      B = 1/4 * C

So, A : B : C = 2 : 3 : 12

A’s share = 2/17 × 510 = 60

B’s share = 3/17 x 510 = 90

C’s share = 12/ 17 x 510 = 360

20. (c); Let Boys = 3x and girls = 2x

No. of students who do not get scholarship = (80% of  3x) + (75%  of  2x) = 39x/10

Req. percentage = (39x/10 × 1/5x × 100)% = 78%

21. (b); 10% of B = 1/4 ×G

B/G = 5/2

22. (b); Let fixed amount = x

Cast of each unit = y

540y + x = 1800

620y + x = 2040

from (i) and (ii)

x = 180,  y = 3

So, total charges for consuming 500 units = (180 + 500 * 3) = 1680

23. (b); Let C gets Rs. x and B gets Rs. (x + 8)

A gets Rs. (x + 15)

So, Sum of A + B + C = 53

x + x + 8 + x + 15 = 53

x = 10

So, A: B: C = 25 : 18: 10

24. (c); Let the no. of 25p, 10p and 5p coins be x, 2x and 3x respectively.

So, Sum of their values = (25x/100 + (10 * 2x)/100 + (5 * 3x)/100) = 60x/100  = 30

So, x = 50

So, the no. of 5p coins = 3 x 50 = 150

Shortcut:

                          25p      10P        5P

No. of coins      1      :   2      :     3 

=300/6

So, No. of 5p coins = 50 × 3 = 150 coins

25. (b); From the question

50 * 2K + 100 * 3K + 500 * 4K + 1000 * K = 34000

K = 10

So, No. of 500 notes = 4 × 10 = 40

26. (a); From the Question C/(A + B) = 3/4,        B/(A + C) = 12/23

So, the sum of both term of the ratio C + A + B = 3 + 4 = 7

So, runs scored by C= 3/7 × 1750 = 750 runs

Similarly,

runs scored by B = 12/ 12+23 × 1750 = 600 runs

and runs scored by A = 1750 – 750 – 600 = 400run

27. (c); No. of employees in class – 1 = 5/17 * 68 = 20

No. of employees in class – 2 = 10/17 * 68 = 40

No. of employees in class – 3 = 2/17 * 68 = 8

So, Salary of each class employee be 2x, 3x and 5x.

So, 20 * 2x + 40 * 3x + 8 * 5x = 400000

x = 2000

So, salary of employee of class – 3 is = 10000

28. (a); According to the Question 20 * 2x + 40 * 3x + 8 * 5x = 400

x = 2

So, he spent 20 * 2 * 2,     40 * 3 * 2,      8 * 5 * 2 on those three vehicles respectively.

So, the spent on car = 240

29. (a); Price ∝ (weight)²

P ∝ W²

P = K * (10x)²  ………… (i)

Now, total price of broken pieces of diamond P_n = K[x² + (2x)² + (3x) ² + (4x)²] = 30K * x² ……….(ii)

A.T.Q.

P – P_n = 2, 10,000

K * 100x² – K * 30x² = 2, 10,000

70K * x² = 2, 10,000

Price of unbroken diamond = 100K * x² = 3 ,00,000

30. (a); (a + b)/5 = (b + c)/6 = (c + a)/7 = (a + b + b + c + c + a)/(5 + 6 + 7)

= 2(a + b + c)/18

a + b = 5x,    a + b + c = 9x,   b + c = 6x and c + a = 7x

Now, a + b + c = 18

So, the value of x = 18/9 = 2

So, b = 2 * 2 = 4

31. (a); 2a = 3b

a/b = 3/2,       b/c = 4/3

So, a:b:c = 6:4:3

32. (a); The ratio of 1 rupees: 50 paise: : 25 paise = 5/6 × 3/ 5 : 3/5 :1 

= 5:6:10

From the Question

5K + 50/100 * 6K + 25/100 * 10K = 262.5

K = 25

So, the no. of 25 paise coin is = 250

33. (a); male amount/ female amount = 4/3  and female amount/ children account = 6/5

So, ratio of male amount: female amount: children = 8:6:5

from the question,

10 * 8K + 15 * 6K + 5 * 5K = 11700

K = 60

So, each child get = 300

34. (a); From the question

(5x – 2500)/(4x – 2500) = 10/7

x = 1500

Shyam’s income = 4 × 1500 = 6000

35. (a); 1 cows = 3 calves

The ratio of the cattles of A, B and C.

= 4×3+3:5×3+4:6×3+5 = 15: 19: 23

Ratio of rents = 15×5:19 × 3:23 × 2 = 75: 57: 46

Rent of B = 2670 * 57/ 75 + 57 + 46 = 855

The difference between rent of A and C = (75 – 46)/(75 + 57 + 46) * 2670 = 435

36. (a); The ratio of capitals of OM, Jai, Jagdish

= 5000 × 12:3000×4+4000×8:7000×8+3000 × 4 =  15: 11: 17

So, Jagdish’s share = 17/43 × 34400 = 13600

37. (b); Let Ram, Krishna and Ganesh invested 5x, 4x and 3x.

Ram’s Capital = 5x * 3 + 3x * 6 = 33x

Krishna’s Capital = 4x * 4 + 3x * 5 = 31x

Ganesh’s Capital  = 3x * 4 + 4x * 5 = 32x

 So, clearly shows Max. profit gained by Ram.

Ram’s profit = 33/96 × 19200 = 6600

38. (c); The amount given to B as management fees = 12%  of 7500 = 900

the rest amount of profit = 7500 – 900 = 6600

Ratio of capitals of A and B = 12000/ 14400 = 5/6

A’s share = 5/ 5+6 × 6600 = 3000

B’s share = 7500-3000 = 4500

So, Difference between A and B share is = 1500 

39. (a); Let the capital of Laxmi and Saraswati be 7x and 5x.

Let, Saraswati’s capital was invested for y months.

So, the ratio of their capital = 7x × 20 : 5x × y

140x/ 5xy = 4/3 ,   y = 21 months

40. (c); p/q = r/s = t/u = 2/3 ⇒ mp/mq = nr/ns = ot/ou = 2/3

⇒ (mp + nr + ot)/(mq + ns + ou) = (2 + 2 + 2)/(3 + 3 + 3) = 2/3

41. (a); √2 / (1+√3) = √6/ x 

x = √3 (1+√3)  = 3 + √3

42. (d); Ratio of Vinay and Aditya for one month

= (50000 × 12) + (80000 × 24): (70000 × 24)

= 600000 + 1920000 : 1680000 = 3:2

Hence, share of Aditya in the profit earned from the business = 87500/ (3+2) x 2 = 35000

33. (b); Let the score of Ajay = x

Rahul = x-15

Manish = x – 25

As per the question

x = 63 + 30 = 93

score of Ajay = 93

Rahul = 93-15 = 78

Manish = 93-25 = 68

Total marks of Rahul, Manish and Suresh = 3 * 63 = 189

Suresh = 189 –  (78 + 68) = 43

Manish + Suresh = 68 + 43 =111

44. (d); Let A’s amount be 5x

B’s amount be 6x

Again let B invested the capital for y months.

5x × 8/ 6x × y = 5/9 ⇒ 40/ 6y = 5/ 9

y = 9 × 40/ 6 × 5 = 12 months 

45. (d); Ratio of profit among A, B and C

= (42000 × 4 +30000 × 6) : (30000 × 4 + 24000 × 6) : (28000 × 4 + 20000 × 6)

= 348 : 264 : 232

Hence C’s share = (46420/844) × 232 = 12760

46. (c); Difference of amount received by R and Q is (7 -5) = 2

Total amount received P and Q = (3 + 5) = 8.

Then, 2 corresponds to 4000 implies that 8 corresponds to (4000/ 2) × 8 = 16000

47. (d);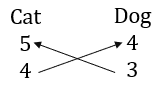 

48. (a); Total of max. marks of all subjects = 105 × 5 = 525

80% of 525 = (525 × 80)/ 100 = 420

Obtained marks of 4 subjects = 360

So, marks obtained in science = 420-360 = 60

49. (c); There are 70 males out of 120 applicants, there must be 50 females.

Thus, no. of males having a drives’s licence all 50 females must having a drives’s licencse will be 80-50 = 30. The max. possible no. of males having a driving license is 70.

So, ratio between the min. and max. is 30:70 or 3 :7.

50. (b); Given ratio of employees = 9:13:18

A.T.Q.

18x = 54

x = 3

No. of employees of type A = 9 × 3 = 27

Similarly, wages of every employee of type A = (10/7) × 1400 = 2000

Req. wages = 27 × 2000 = 54000